What are the shortest
paths on polyhedron?
A polyhedron is a solid
bounded by a finite number of polygonal plane faces and, if 2 points lie on the
same face, the shortest path is the straight line segment. If instead the 2
points lie on adjacent faces, then the shortest path belongs to a straight line
on the developed surface (open on the plane).
Prolonging this
segment, beyond its extremes, we can join all the pairs of points on the
surface of the polyhedron.
A cubic-edged planet
with an edge equal to the diameter of
the Earth (12,746 km) would have a diagonal of 22,077 km. The difference
between the highest and the deepest point would be 4,665 km (4,665,000 meters).
On Earth between Mount Everest (8,844
m) and the Mariana Trench (-10,911
m) there is a difference in height of 19,755 m. Assuming 10,000 m deep oceans,
the 8 vertices would be mountains 500 times the height of Mount Everest. To go
from one of the 6 faces to the other you should overcome passes with an
altitude equal to 300 times Mount Everest. The oceans could be at most 6.
On the Carlos
Furuti website, you can find many Cartographic
Projections:
and you can even print a cubic Earth.
The great British mathematician Henry Ernest Dudeney (1857 - 1930) invented hundreds of
mathematical games a couple will be reported dealing with geodesics on
polyhedron.
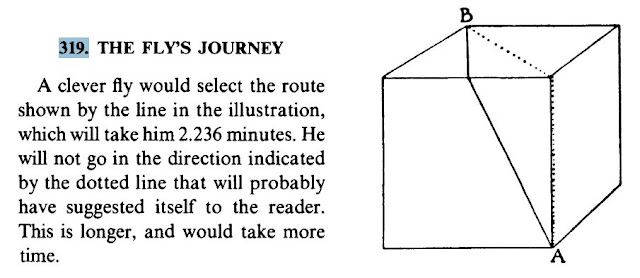
THE
JOURNEY OF THE MOSCOW
A fly, starting from point A, can travel the 4 sides of the base of a cubic block in 4
minutes. How long will it take to get from A
to the opposite corner B?
The
fly would select the path shown by the line in the illustration, which will
require 2.236 minutes. It will not go in the direction indicated by the dotted
line that might seem the suggested one. This path is longer and takes more time
(2.414 minutes).
THE SPIDER AND THE FLY
A rectangular room has
the dimensions shown in the figure (in English measurements). A spider,
indicated with the yellow star, is located in the center of one of the two
bottom walls one foot from the ceiling. A fly, indicated with the dark star, is
instead found on the opposite wall, one foot from the floor. What is the
shortest distance the spider has to travel to reach the fly?
The easiest way to solve the problem is to develop the
room on the floor. In this way the shortest path is the hypotenuse of a right
triangle, equal to 40 feet.
The paradox, in this case, consists in the fact that the horizontal path might seem shorter, but it is not difficult to verify that it is equal to 42 feet (2 more).
http://mathworld.wolfram.com/SpiderandFlyProblem.html
https://pbbmath.weebly.com/blog/the-logic-puzzles-of-henry-dudeney
http://www.ilovephilosophy.com/viewtopic.php?f=4&t=181293
https://math.stackexchange.com/questions/292495/gentle-introduction-to-quasi-geodesics
http://www.progonos.com/furuti/MapProj/Normal/ProjPoly/projPoly3.html



No comments :
Post a Comment